Science Assessment ~ Items ~ RG179001

A person drops a rock off of a cliff, which is 100 meters high. As the rock falls, its speed increases, and its mass stays the same.

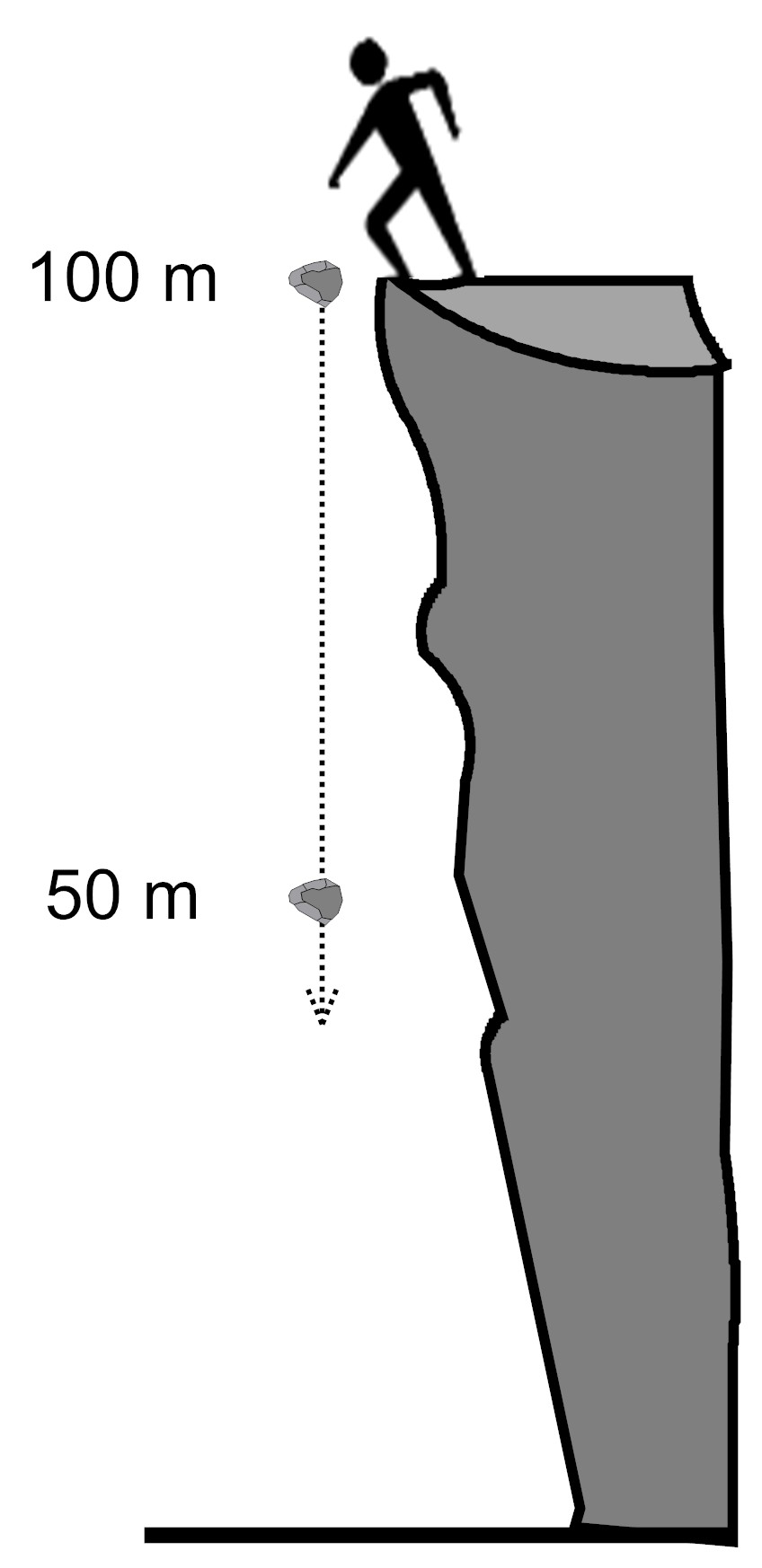
How does the gravitational potential energy of the rock change from the point at which the person drops it to the point at which it is 50 meters above the ground?

The gravitational potential energy of the rock at 50 meters is double the gravitational potential energy at 100 meters because the amount of gravitational potential energy an object has is inversely proportional to the height the object is above the ground.
The gravitational potential energy of the rock at 50 meters is half of the gravitational potential energy at 100 meters because the amount of gravitational potential energy an object has is directly proportional to the height the object is above the ground.
The gravitational potential energy of the rock at 50 meters is the same as the gravitational potential energy at 100 meters because the amount of gravitational potential energy an object has depends only on the mass of the object, and the mass stayed the same.
The gravitational potential energy of the rock at 50 meters is zero because gravitational potential energy is the potential to fall; therefore, the rock lost its gravitational potential energy when it was dropped.

Details
Performance
NGSS

Topic
Correct Answer: B
Misconceptions

Distribution of Responses

Chart showing distrubtion of responses for Item 179001

Scale Score for Item Difficulty (200[Easy]-800[Difficult]): 518

Students Responding Correctly

Group	Correct	Total	Percent
Overall	2291	7092	32%
Grades
4–5	N/A	N/A	N/A
6–8	1234	4092	30%
9–12	1057	3000	35%
Gender
Male	1113	3397	33%
Female	1128	3541	32%
Primary Language
English	1985	6131	32%
Other	248	769	32%

View data table

Disciplinary Core Ideas: PS3.A A system of objects may also contain stored (potential) energy, depending on their relative positions.

Science Assessment

Item RG179001: The gravitational potential energy of a falling rock at 50 meters is half of the gravitational potential energy at 100 meters because the amount of gravitational potential energy an object has is directly proportional to the height the object is above the ground.